| 22 |
Connecting math domains for new discoveriesSeries expansion and structure theorem on automorphic forms 
|
|---|
Fermat's last theorem is one of the most well-known theorems in mathematics. It was stated by Fermat over 300 years ago and solved by Wiles in 1995. In his proof, the theory of modular forms, which connects various areas of mathematics, such as algebra, geometry, and analysis, plays a significant role. Several conjectures are solved via modular forms, for example, the hypersphere packing problem for 8 and 16 dimensions, and the Moonshine conjecture. However, only a small class of modular forms are used in proofs. These modular forms are called holomorphic. To obtain more applications of modular forms, it is important to analyze the non-holomorphic modular forms. In this study, we determined a method of constructing and analyzing a certain class of non-holomorphic modular forms. On the basis of this research, we will conduct more mathematical investigation and hope to contribute to major conjectures of mathematics such as Langlands conjecture.
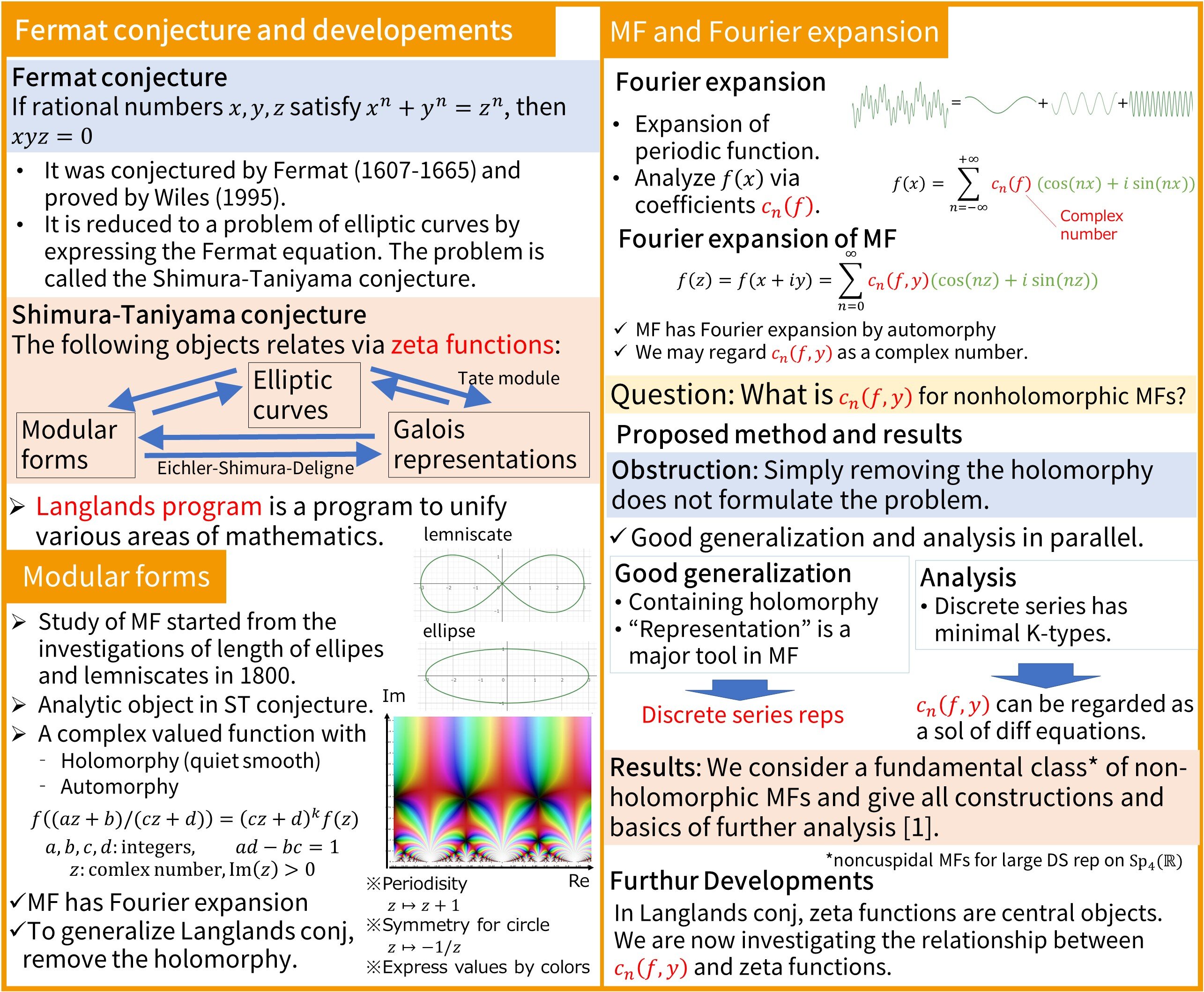
[1] S. Horinaga, H. Narita, “Cuspidal components of Siegel modular forms for large discrete series representations of 
,” manuscripta mathematica, pp. 1-44, 2023
Shuji Horinaga
Computing Theory Research Group, Media Information Laboratory / Institute for Fundamental Mathematics